Chemistry: Limiting Reactant Problems
Limiting Reactant Problems
Now that you're a pro at simple stoichiometry problems, let's try a more complex one.
Using the recipe for ice water (1 glass of water + 4 ice cubes = 1 glass of ice water), determine how much ice water we can make if we have 10 glasses of water and 20 ice cubes.
Hopefully, you didn't have too much trouble figuring out that we can make only five glasses of ice water. Let's go through this calculation carefully to see what we did (it'll be clear why we need to do this in a second).
Molecular Meanings
The limiting reactant in a stoichiometry problem is the one that runs out first, which limits the amount of product that can be formed. The other reactant is called the excess reactant.
- Using our recipe, we can make 10 glasses of ice water with 10 glasses of water.
- With the same recipe, we can make 5 glasses of ice water with 20 cubes of ice.
- Because we run out of ice before we run out of water, we can only make five glasses of ice water. There will be five glasses of warm water left over.
In our example, we would say that ice is the limiting reactant. The ice is said to be "limiting" because it is the ingredient we would run out of first, which puts a limit on how much ice water we can make. The water is called the excess reactant because we had more of it than was needed.
Limiting Reactants in Chemistry
We can use this method in stoichiometry calculations. Again, if we're given a problem where we know the quantities of both reactants, all we need to do is figure out how much product will be formed from each. The smaller of these quantities will be the amount we can actually form. The reactant that resulted in the smallest amount of product is the limiting reactant. Let's see an example:
Example: Using the equation 2 H2(g) + O2(g) ↔ 2 H2O(g), determine how many moles of water can be formed if I start with 1.75 moles of oxygen and 2.75 moles of hydrogen.
You've Got Problems
Problem 2: Using the following equation, determine how much lead iodide can be formed from 115 grams of lead nitrate and 265 grams of potassium iodide:
Pb(NO3)2(aq) + 2 KI(aq) ⇔ PbI2(s) + 2 KNO3(aq)
Solution: Do two stoichiometry calculations of the same sort we learned earlier. The first stoichiometry calculation will be performed using "1.75 mol O2" as our starting point, and the second will be performed using "2.75 mol H2" as our starting point.
- 1.75 mol O2 × 2 mol H2O⁄1 molO2 = 3.50 mol H2O
- 2.75 mol H2 × 2 mol H2O⁄2 mol H2 = 2.75 mol H2O
Because "2.75 mol O2" is the smaller of these two answers, it is the amount of water that we can actually make. The limiting reactant is hydrogen because it is the reactant that limits the amount of water that can be formed since there is less of it than oxygen.
How Much Excess Reactant Is Left Over?
You've Got Problems
Problem 3: Using your results from problem #2 in this section, determine the amount of excess reactant left over from the reaction.
Once we've determined how much of each product can be formed, it's sometimes handy to figure out how much of the excess reactant is left over. This task can be accomplished by using the following formula:
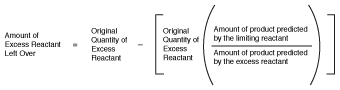
Figure 19.2Formula for finding out how much excess reactant is left over.
In our limiting reactant example for the formation of water, we found that we can form 2.75 moles of water by combining part of 1.75 moles of oxygen with 2.75 moles of hydrogen. Because hydrogen was the limiting reactant, let's see how much oxygen was left over:
- O2 = 1.75 mol O2 - (1.75 mol O2)
(2.75 mol/3.50 mol) remaining
= 0.375 mol O2 remaining

Excerpted from The Complete Idiot's Guide to Chemistry © 2003 by Ian Guch. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.
To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.
