Geometry: Ratio, Proportion, and Geometric Means
Ratio, Proportion, and Geometric Means
Before you start analyzing similar triangles, you need to pick up a few more algebraic supplies. You'll be dealing with fractions and ratios a lot in this section, so you might as well brush up on those algebra skills first.
A ratio is a quotient a/b, where b ≠ 0. A ratio provides a comparison between the numbers a and b. For example, if a is twice as big as b, then the ratio a/b is 2/1. The ratio a/b is read “a to b” and is sometimes written in the form a:b.
There are times when you might want to compare three or more items. When that happens, a simple fraction just won't cut it. You'll need to use what is called an extended ratio. An extended ratio is written in the form a:b:c (if you are comparing three quantities) or a:b:c:d (if you are comparing four quantities). If you are comparing lots of quantities, just keep adding them on, separating each quantity with a colon.
Eureka!
An extended ratio compares more than two quantities and cannot be expressed as a single fraction.
A proportion is a statement that two ratios are equal. The proportion a/b is read as “a is to b as c is to d.” The first and last terms (a and d) are called the extremes, and the middle terms (b and c) are called the means. There are several useful properties involving proportions, and these properties can be established using algebra.
- Property 1: The Means-Extremes Property. In a proportion, the product of the means equals the product of the extremes. That is, if a/b = c/d (where b ≠ 0 and d ≠ 0), then a · d = b · c.
This is just an old-fashioned “cross-multiply” step used in algebra to get rid of unwanted denominators when dealing with fractions. You can use the Means-Extremes Property to solve algebraic equations.
- Example 1: Use the Means-Extremes Property to solve for x: x + 1/9 = x - 3/3.
Solution: If we apply the Means-Extremes Property to our equation, we have
3(x + 1) = 9(x − 3)
3x + 3 = 9x − 27
6x = 30
x = 5
Example 2: Use the Means-Extremes Property to solve for x: 4/x = x/9.
Solution: If you apply the Means-Extremes Property to your equation, you have
36 = x2
x = ± √ 36 = ±6.
Now combine these ideas with a problem involving geometry.
- Example 3: Suppose that two complementary angles are in the ratio 2 to 3. Find the measure of each angle.
- Solution: Let one of your angles have measure x and the other angle have measure y. Because your two angles are complementary, x + y = 90º, so y = 90º − x. Because the ratio of the angle measurements is 2 to 3, you have the following proportion:
- x/y = x/90º − x = 2/3
- You can then use the Means-Extremes Property:
- 3x = 2(90º − x)
- 3x = 180º − 2x
- 5x = 180º
- x = 36º
Now that you know the measure of one of your angles, you can find the measure of the second angle because the two angles are complementary:
- y = 90º − x = 90º − 36º = 54º
There are other properties of proportions. You can flip the proportions and mix and match numerators and denominators. Property 2 of proportions provides a list of the changes you can make.
- Property 2: In a proportion, the means or the extremes (or both the means and the extremes) may be interchanged. That is, if a/b = c/d and a, b, c, and d are all non-zero, then a/c = b/d, d/b = c/a and d/c = b/a.
Again, mixing numerators and denominators is not surprising because you've been doing this in algebra for years! But there is another property of proportions that might be a bit surprising. You have to be careful when you apply this property because it involves adding or subtracting things.
- Property 3: If a/b = c/d, where b ≠ 0 and d ≠ 0, then a + b/b = c + d/d.
To derive this property, start with a/b = c/d, add 1 to both sides and add the fractions.
Now that you have proportions under your belt, you can talk about the geometric mean of two numbers. If you start with a proportion where the two means are identical (and the two extremes may be different), such as a/b = b/d, then b is the geometric mean of a and d. You found the geometric means of the numbers 4 and 9 in Example 2. Although a pair of numbers actually has two geometric means (one positive and the other negative), geometers are only interested in the positive one. After one more example you'll be ready to apply these algebraic properties to geometry.
- Example 4: In Figure 13.1, suppose that AD is the geometric mean of BD and DC. If BC = 13 and BD = 9, find AD.
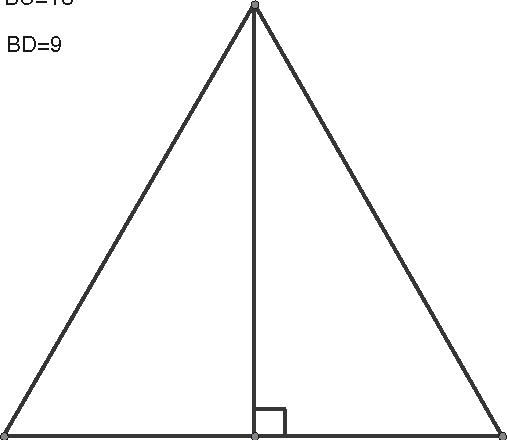
Figure 13.1AD is the geometric mean of BD and DC, BC = 13 and BD = 9.
- Solution: Because AD is the geometric mean of BD and DC, You know that BD/AD = AD/DC. You are given that BD = 9, but you still need to find DC. Using the Segment Addition Postulate, BC = BD + DC. Substituting in for BC and BD gives 13 = 9 + DC, or DC = 4. Now you can substitute the values into the proportion and solve for AD:
- BD/AD = AD/DC
- 9/AD = AD/4
- (AD)2 = 36
- AD = 6

Excerpted from The Complete Idiot's Guide to Geometry © 2004 by Denise Szecsei, Ph.D.. All rights reserved including the right of reproduction in whole or in part in any form. Used by arrangement with Alpha Books, a member of Penguin Group (USA) Inc.
To order this book direct from the publisher, visit the Penguin USA website or call 1-800-253-6476. You can also purchase this book at Amazon.com and Barnes & Noble.
